Mechanical Systems

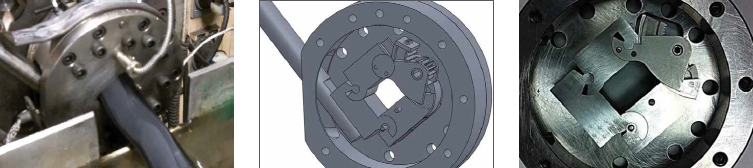
The lifeblood of manufacturing development hinges on increasing production, lowering costs and deftly overcoming any engineering problems that may arise. The backbone of any industrial facility is the mechanisms by which products are produced.

Andrew Murray, Ph.D., a professor in the University of Dayton’s Mechanical & Aerospace Engineering Department, and David Myszka, an associate professor, are using the Ohio Supercomputer Center’s high performance computing resources to investigate techniques for designing better machines to help manufacturers continue to become more efficient.

“The goal is improved algorithms for the synthesis of mechanical systems like what we see in scissor jacks, dump trucks, folding lawn chairs, for example, these mechanisms are incredibly common,” Murray said. “And in many cases, you don’t need a fancy design process to solve the problem, engineers do it every day. But to push into new areas and try to design their new machines, we need to develop the corresponding mechanism design algorithms.”

Murray and his research team are working toward creating a novel approach to designing three-dimensional spatial linkages and analyzing their kinematic output—the kinematics is essentially the motion you want a system to have. To do that, they have to tackle the intense mathematics associated with the design analysis.

Many of the designs within the machines manufacturers are described by a collection of three components: Revolute Joints (hinge or pin joints), Prismatic Joints (sliders or pistons), and Rigid Bodies (links or connectors).

“The kinematics are typically described by algebraic equations,” Murray said. “As we write these equations for increasingly complicated systems, the mathematics explode. It’s beyond our capacity. But we’re using supercomputers to help do the problems that are building to that.”

Murray’s method is first to construct a mathematical model for spatial linkages. He then uses OSC’s Oakley Cluster for a software package called Bertini, which requires parallel computation. Matlab analyzes the results. The results are then validated through physical models.

“Bertini is beautiful because it starts with a simple version of your problem and grows from that,” Murray said. “Because it grows, it tracks every solution possible to that system of equations.

“We don’t just get to look at what we have via optimization, we get to look at everything. And because these systems have so many solutions, there can be a solution sitting right next to the one you optimized that may be better.”